Examples of path function: Change in heat and work done.
Properties of a system are of two types:
i. Intensive properties and ii. Extensive properties
i. Intensive properties: Properties of the system which are independent of the mass of the system are called the intensive properties.
Example: Pressure, Temperature, vapour pressure, molar volume, density, viscosity, surface tension, refractive index, specific heat, concentration like normality, Molarity etc., standard emf of cell etc.
ii. Extensive properties: Properties of the system which depend on the mass of the system are called the extensive properties.
Example: volume , Mass, internal energy, enthalpy etc.
Note: The ratio of two extensive properties give an intensive properties.
Example: Pressure = Force / Area, Density = Mass / Volume
Extensive properties specified per gram or per mole such as specific heat becomes an intensive properties.
Extensive properties are additive in nature whereas intensive are not.
Thermodynamic Equilibrium: When the observable properties of a system do not change with time the system is said to be in thermodynamic equilibrium.
A system is said to be in thermal equilibrium if the temperature is same throughout system.
When composition of the system does not vary with time then it is said to be in a chemical equilibrium.
When there is no macroscopic movement within the system then the system is said to be in mechanical equilibrium.
Thermodynamic process: The path or operation by which a thermodynamic system changes its state from one to another is called a process.
These are of following types:
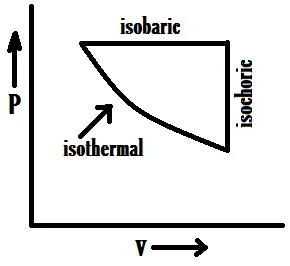
i. Isothermal Process: The process in which temperature of the system remains constant throughout is called an isothermal process.
i.e., dT = 0
Thus heat can be exchanged between the system and surroundings in order to maintain the temperature of the system constant.
Example: During freezing the temperature remains constant both for liquid and solid. Similarly melting, evaporating, condensing etc.
ii. Adiabatic Process: The process in which there is no exchange of heat between the system and surroundings is called an adiabatic process.
i.e., dq = 0
Since heat exchange is not possible between the system and surrounding, the system uses its internal energy for work done.
iii. Isobaric Process: The process in which pressure of the system remains constant throughout is called an isobaric process.
i.e., dP = 0
Heating water at its boiling point keeps the pressure constant. Thus its an isobaric process.
iv. Isochoric Process: The process in which volume of the system remains constant throughout is called an isobaric process.
i.e., dv = 0
Carrying out reaction in a fixed and rigid container is an isobaric process.
v. Cyclic Process: The process in which the system regains its initial state after a series of different steps is called a cyclic process.
In this process many of the thermodynamic functions remains constant like, dU = 0, dH = 0, dG = 0, dT = 0, dp =0, dV = 0 but the work done and heat (i.e., dw and dq ) being path function do not become equal to zero.
vi. Reversible Process: The process which occurs infinitesimally slowly and can be reversed by an infinitesimal change in the thermodynamic parameters is called a reversible process.
Example: Slow isothermal expansion of gasses
vii. Irreversible Process: A process in which the initial state of the system spontaneously changes into the final state, in a single step, is called the irreversible process.
Example: Propane on combustion produces CO2 and H2O which do not react back to give propane again.
Internal Energy: The tem internal energy in thermodynamics means the total energy stored inside a system. The sum total of kinetic and potential energy of the molecules of the system is referred to as internal energy (U).
We actually measure the change in internal energy of the system not the absolute internal energy.
Thus the change in internal energy = Final internal energy - Initial internal energy
=> ΔU = Uf - Ui
Work done in irreversible isothermal expansion:
If the internal pressure (the
pressure of the gas) is much larger than the external pressure, the gas expands
in a single step until it reaches mechanical equilibrium. That means the
internal and external pressure becomes equal. This is called an
irreversible process. If the temperature remains constant throughout, the
expansion of the gas is called the irreversible isothermal expansion, the work done is called the expansion work. The gas
expands against the external pressure (force). Thus work done is determined
according to external pressure.
Work = F.S Cos θ = Force X Displacement X Cos θ
Work done by the system = Force X Displacement X Cos 1800
(When gas expandsExpansion work) on the surrounding
(The work is done against constant external pressure.)
P(external) = Force/Area => Force = P(external) X Area
Work done by the system on the surrounding = Force X Displacement X Cos 1800
If dl is the infinitesimal small distance moved by the piston, then the small work done,
δW = - F.dl = - P(external) X Area X dl = - P(external) dV
The total work done in changing the volume from V1 to V2 = W = - P(external) ΔV
=> Work done by the system = - P(external) (V2 - V1) Ltr atm
The unit of work done is in ltr atm which must be converted into joule by applying conversion factor, 1 ltr atm = 101.32 joule
Sign Convention:
Work done by the system (Expansion work, V2>V1) = Negative
Work done on the system (Compression work, V2<V1) = Positive
In a similar way,
Heat lost by the system = Negative
Heat gained by the system = Positive
Change
in enthalpy:
From
first law of thermodynamics,
ΔU = q – w = q - P ΔV --------- eq. 1
Suppose we supplied heat but did not allow the
volume of the system to change, then, ΔV = 0. => P ΔV = 0
=> ΔU = qv
This means heat supplied to a system at constant
volume is equal to the change in internal energy or in other words heat
supplied to a system at constant volume increases the internal energy of the
system.
Again rearranging the equation 1, we have,
q = ΔU + P ΔV
Suppose we supply heat to the system at
constant pressure. This time there will definitely be a change in internal
energy of the system and the system will do some work.
The thermodynamic property U + PV is called
the heat content or enthalpy (H) of the system, and ΔU + P ΔV is called the change
in enthalpy.
Thus qp = ΔH = ΔU + P ΔV
Hence the change in enthalpy of the system is defined
as the amount of heat absorbed at constant pressure provided that only work
done is of pressure volume type.
Sign Convention:
ΔH is +ve if heat is absorbed by the system
and is –ve if lost by the system.
The Second Law of Thermodynamics:
Limitations of the First Law:
1. The first law only gives the relationship between the heat supplied and the work done by a system in any given process, but it does not confirm the direction of flow of heat. That means, whether heat can flow from a cold body to the hot one.
2. According to first law, the energy of an isolated system remains constant during a specified change or process, but it is unable to predict whether the change or process is spontaneous. (A spontaneous process is one which occurs by its own accord and does not need any driving force). The first law does not tell whether a gas can diffuse from low pressure to high pressure or whether water can run uphill etc.
3. The first law indicates that heat can be converted into work but it does not inform that whole of the heat supplied to a system can not be converted into work.
All the above made points suggest that there is a need of another law of thermodynamics. The law that can further explain all the above points is known as The Second law of Thermodynamics. Before we discuss the statement of the second law, we must understand the spontaneity of a process and how to relate it with thermodynamical functions like enthalpy, entropy and Gibb's free energy.
Spontaneous and Non-spontaneous Processes:
The process which occurs by its own accord under some given conditions or by initiation is called a spontaneous process. These process once started proceed to the finish unless there is any outside intervention.
Example: 1. Two moles of a gas at 25 degree centigrade and 2 atm pressure is allowed to expand to a greater volume keeping temperature constant.
2. Dissolution of sugar in water.
3. Burning of coal in presence of oxygen.
4. Diffusion of tea as the tea bag is dropped into hot water.
5. Water running downhill.
Some spontaneous processes need initiation, and once initiated they continue to flow. burning of coal needs initiation (to bring the flame near the coal).
A process which is spontaneous is also called feasible.
Non-spontaneous processes are those which can neither take place by their own accord nor by initiation.
Example: 1. Diffusion of a gas from low pressure to a high pressure.
2. Dissolution of sand in water.
3. Flow of heat from cold body to hot one.
4. Combination of water and carbon dioxide to form methane.
Direction of spontaneous change and the driving force:
The force which is responsible for the spontaneity of a process or which drives the process or a reaction in certain direction is called the driving force.
Spontaneity and Enthalpy (Tendency for minimum or lowering of energy):
For many spontaneous processes we can observe that the direction of change is towards the lowering of energy. That means in these spontaneous processes energy is lost. We indicate this by writing with a negative value of enthalpy (for example, ΔH = -50 J, because heat lost from the system is negative).
For example, when acids and bases are added together they react spontaneously and release energy and warm up the container.
When nitrogen and hydrogen gases react they form ammonia spontaneously and energy is produced or lost from the mixture or system.
N2 (g) + 3H2 (g) ======> 2NH3 (g) + 92.2Kj per mole
Thus the first factor which is responsible for the spontaneity of a process is the tendency to acquire minimum energy or lowering of energy (accompanied by a negative enthalpy value).
But we can not conclude that for all the spontaneous processes energy is lost. This is because there are some exceptional spontaneous processes in which energy is gained. For example when ammonium chloride is added to a test tube containing lukewarm water it cools down. This indicates that energy has been absorbed as the salt dissolved spontaneously. There are several similar exceptional cases, called the endothermic reactions where energy is gained ( ΔH = positive) but still they are spontaneous.
Thus it is not possible to determine the spontaneity ( whether spontaneous or nonspontaneous) of a reaction or process from the magnitude and sign (whether positive or negative) of change in enthalpy. Therefore we need another thermodynamical function to determine the spontaneity of a process or a reaction.
Spontaneity and Entropy (Tendency for maximum randomness):
The exceptional case of dissolution of ammonium chloride in water as discussed above in which energy was being gained (ΔH = positive though process was spontaneous) can be explained using the tendency to acquire maximum randomness. That means the ions of the ammonium chloride as it dissolved gets distributed through out the solution which indicates that the system (or the solution) has a greater extent of disorder or randomness.
We can take take another example. Consider two different gasses are contained in tow different bulbs connected through a narrow tube separated by a stop-cock. When the stop-cock is opened mixing of gases takes place spontaneously. In this case also there is an increase in the randomness or extent of disorder as the gases mix up.
Thus the second factor which is responsible for the spontaneity of a process is the tendency to acquire maximum randomness.
The thermodynamic function used to express the extent of disorder or randomness in a system is called the entropy (S). Higher is the randomness of a system higher is the entropy.
For example we can consider solid ice, liquid water and water vapour. As there is almost regular arrangement of water molecules solid ice the entropy in this case is lowest and accordingly highest in water vapour.
The change in entropy (ΔS) is proportional to the amount of heat (q) absorbed isothermally and reversibly (infinitesimally slowly) and inversely proportional to the absolute temperature at which the heat is absorbed.
Thus ΔS = q(rev)/T
Thus entropy change during any process is defined as the ratio of heat absorbed isothermally and reversibly to the absolute temperature at which the heat is absorbed.
Like all other state functions, change in entropy (ΔS) depends only on the initial and final step. Thus ΔS is also a state function.
ΔS = S2 - S1 = Sum total of entropy of products - Sum total of entropy of reactants
Unit of change in entropy = J per kelvin per mole or Cal per kelvin per mole
The concept of supplying heat to a system reversibly or
infinitesimally slowly to measure the entropy lies in the change in internal energy which is a state function, given by the first law of thermodynamics, ΔU = q+W. The value of change in internal energy remains constant (which depends only on the initial and final state) whatever path may be followed. This further follows that each combination of q and w constitutes a different path between the same initial and final state. We have seen from the reversible expansion of a gas that, greater is the number of infinitesimal small steps greater is the work done. Since in a reversible process we get maximum work done which is the characteristics of that process the heat gained in each small step is at its smallest. Thus the maximum work done Wmax and minimum heat qmin (or the heat change done infinitesimally slowly or reversibly) have unique values for any given process and hence are state functions.
It has been proved that for all spontaneous processes occurring in isolated system the change in entropy is positive [ΔS (System) = +ve].
But there are certain limitations also when we try to determine the spontaneity of a process using the tendency to acquire maximum entropy. For instance, we can take the example of adsorption of gas on metal surface or solidification of liquid water below zero degree centigrade and 1 atmospheric pressure. Though these processes are spontaneous yet the change in entropy of the gas when it is adsorbed on the solid metal surface decreases and similar is case of change in entropy of liquid water. Thus the change in entropy of the gas in this process is negative. The main problem here is that we have not considered the change in entropy of both the system and surroundings. The total entropy change of the system and the surroundings is called the change in entropy of the universe.
ΔS (System) + ΔS (Surroundings) = ΔS (Universe)
For all spontaneous processes, where the change in entropy of both the surroundings and system can be measured, will show an increase in entropy of the universe. i.e., ΔS (Universe) = +ve or ΔS (Universe) > 0
We can demonstrate the above statement by considering the freezing of water. We will consider the freezing of water at two different temperatures, at 0 and -1 degree centigrade. When we consider the freezing of water at 0 degree centigrade the decrease in entropy is calculated by dividing the heat of fusion of ice by the temperature for the reversible pathway,
ΔS (System) = -6000J per mole/273K = -21.978 J per kelvin per mole
Now since the same heat of fusion is transferred to the surrounding at the same temperature, the increase in entropy of the surroundings becomes,
ΔS (Surroundings) = +6000J per mole/273K = +21.978 J per kelvin per mole
So the ΔS (Universe) = ΔS (System) + ΔS (Surroundings) = 0 . This indicates that there is no more change in entropy and no more additional freezing of water. The amount of water that has been solidified is now fixed and the ice and water are now in equilibrium.
Thus for a process at equilibrium, ΔS (Universe) = 0
Let us now consider that, the water is supercooled from 0 degree centigrade to -1 degree centigrade reversibly before it freezes. Since the cooling started at 0 degree centigrade, the decrease in entropy of the system becomes,
ΔS (System) = -6000J per mole/273K = -21.978 J per kelvin per mole
But the surrounding is absorbing the heat of fusion at -1 degree centigrade or 272 K, hence its increase in entropy becomes,
ΔS (Surroundings) = +6000J per mole/272K = +22.059 J per kelvin per mole
Thus the total change in entropy of the universe becomes,
ΔS (Universe) = ΔS (System) + ΔS (Surroundings) = (-21.978 +22.059) J per kelvin per mole = 0.081j per kelvin per mole, which proves the statement we made above that, The overall or total change in entropy or the entropy of the universe must increase in a spontaneous process or ΔS (Universe) > 0
Statement of the second law of thermodynamics:
As like there are several ways to state first law of thermodynamics we can state the second law in a several ways as well.
First Definition: All spontaneous processes are accompanied by the net increase in entropy. Clearly, the total change in entropy of the system and surroundings (or the change in entropy of the universe) is positive.
So far we have analysed that there are two driving forces for a spontaneous process. The first one is the tendency to acquire minimum energy (loosing energy) and the tendency to acquire maximum entropy (increase in entropy) by a system. In both of the cases we have found exceptions. This further implies that, for any spontaneous process, out of the two driving forces, three different cases may arise. (Let us denote the tendency to acquire minimum energy by "minE" and the tendency to acquire maximum randomness by "maxR")
Case - 1: When both minE and maxR favours (energy is lost from the system and entropy of the system increases), then the net driving force is very large and process becomes spontaneous easily.
Case - 2: When minE favours (energy is lost from the system) but maxR
opposes (entropy decreases) and minE > maxR, then the net driving force though small favours the spontaneity of the process.
Case - 3: When minE opposes (energy is gained by the system) but maxR favours (entropy increases) and minE < maxR, then also the net driving force though small favours the spontaneity of the process.
The Entropy and Unavailable energy:
Let us consider a gas enclosed inside a cylinder with a piston. Suppose q amount of heat is absorbed by the gas. By absorbing energy, the molecules of the gas start moving with higher velocities. Some of the molecules which strikes the piston help the gas change its volume so that some work is done. The rest of the molecules which strike on the wall do not contribute to the work done by the gas. They only increase the disorder or randomness of the system.
Thus whole of the heat or energy available to a system can not be converted into work. A part of heat remains unavailable and only increases the randomness. As we know the amount of randomness of a system is known as the entropy, we say that the unavailable energy is related to the entropy as follows.
ΔS = q/T = (part of heat which is not available to be converted into work / T)
=> The unavailable energy = TΔS
The Alternative statement of the second law of thermodynamics (The Planck Statement): It is impossible to construct an engine which will work in a complete cycle and convert whole of the heat into work without producing any change in the surroundings.
Free Energy and the maximum useful work (Work other than mechanical work done):
We have already discussed that all the energy of the system can not be converted into work. The part of the energy which can be converted into useful work is called the available energy and the rest part is known as unavailable energy.
Thus total energy = isothermally available energy + isothermally unavailable energy
The isothermally available energy present in a system that can be converted into useful work is called the free energy or Gibb's free energy
The change in free energy is related to the change in enthalpy and change entropy by the relationship, ΔG = ΔH - TΔS.
This equation can be derived from the first law of thermodynamics. But we must recall that, work done can be classified into work of expansion and work of non-expansion.
Consider any battery from which we get electrical energy or electrical work done. This energy is available to us from the energy of chemicals inside the battery. There is no need of expansion to avail this energy, hence this energy is called the work of non-expansion (Wnon-exp).
The work done due to change in volume of the system is called the the mechanical work done or the work of expansion. Wexp = PΔV
As we derive the equation for the free energy, we must born in our mind that, till now we were dealing with only mechanical work done, hence the form of first law of thermodynamics was, ΔU = q - W = q - PΔV or q = ΔU + W.
But in actual practice the W in the above equation represent the maximum work done which is composed of the work of expansion and work of non-expansion. Thus the first law of thermodynamics is converted to,
q = ΔU + Wmax = ΔU + Wexp + Wnon-exp ........ eq 1
As the pressure is kept constant, the work of expansion, Wexp = PΔV
Thus q = ΔU + PΔV + Wnon-exp = ΔH + Wnon-exp ..... eq 2 (Since, ΔH = ΔU + PΔV )
But we know, ΔS = q(rev)/T
=> q = TΔS
Substituting q in in eq 2 we get,
TΔS = ΔH + Wnon-exp
=> -Wnon-exp = ΔH - TΔS
=> ΔG = ΔH - T
ΔS (Under constant temperature and pressure)
Notice that, we have written ΔG in place of -Wnon-exp. This indicates that the free energy is a measure of work other than the work of expansion. This work done is called the useful work. For example, in galvanic cell the electrical (work done) energy is obtained, from the free energy which is equal to the product of charge and voltage.
=> - ΔG = nFEcell ,
Where Ecell = EMF of the cell
Spontaneity and free energy:
Without any exception, when ΔG is negative the process is spontaneous, when ΔG is positive the process is nonspontaneous and when ΔG is equal to zero the process is at equilibrium.
Effect of temperature on the spontaneity of a process:
To understand this concept let us explain the following two statements.
1) An endothermic (ΔH = +ve) reaction which may be non-spontaneous at low temperature may become spontaneous at higher temperature.
If the temperature is high then TΔS will be much greater than ΔH in magnitude, making ΔG highly negative and the process spontaneous
2) An exothermic (ΔH = -ve) reaction which may be non-spontaneous at higher temperature may become spontaneous at low temperature.
If the temperature is low so that TΔS will be smaller than ΔH in magnitude, making ΔG negative and the process spontaneous.
To be Continued ...





I admire this article for the well-researched content and excellent wording. Read more info about Reliable Hot Water Services Melbourne. I got so involved in this material that I couldn’t stop reading. I am impressed with your work and skill. Thank you so much.
ReplyDeleteThe subject of physics known as thermodynamics studies how heat, work, and temperature relate to energy, radiation, and the physical properties of matter. It specifically discusses how thermal energy is changed into or out of other types of energy, and how this process affects matter. Many students are looking for important notes on various physics topics, so the notes you shared in your blog were quite beneficial. I recently read in your blog that you illustrate this topic with equations and graphics. Each physics topic can only be thoroughly explained by an excellent physics tutor. Thank you for sharing interesting and valuable information.
ReplyDeleteThank you so much
ReplyDeleteSir I am Satya Prakash Mohanty now in Aryan advance academy group 2 . I feel very lucky for visiting your website
ReplyDelete